The figure shows the basic optical geometry. A collimated laser beam (left) illuminates particles between the 2 windows. A multi-ring detectorplaced at the focal plane of the receiving lens senses the scattered light. The figure also illustrates the Fourier transform property of a lens, namely that any ray originating in water at an angle q reaches the detector at a radius equal to fq.

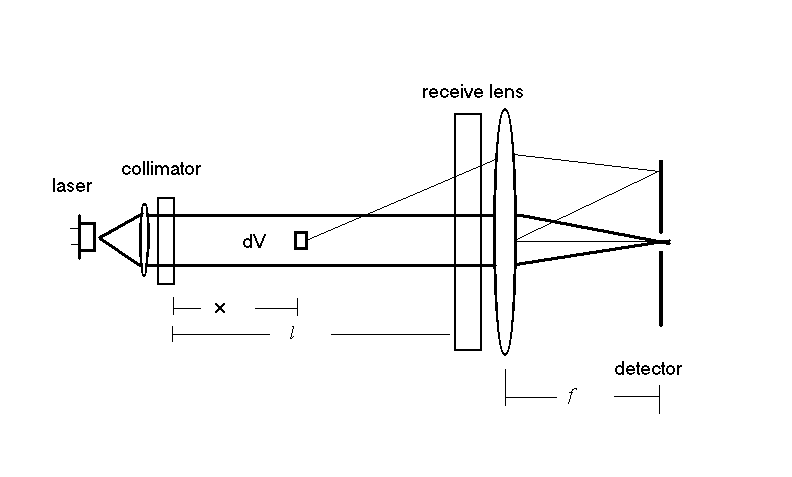
Now, consider a small length dx of the beam, located at a distance x from the transmit window. An elementary volume in the laser beam is dV=dAdx where dA is the area of the elementary volume. Let b(q) be the VSF. Let the optical path of the beam between the windows be l. For optical power P0 entering from the transmit window, and with a beam attenuation coefficient c, by definition of the VSF scattered power in any direction q will be:
dP = e-cxP0/Ab(q)dAdxdW

where dΩ is the elementary receiver solid angle. Further attenuation of scattered light occurs by a factor c-c(l-x) before reaching the window1. The solid angle dΩ is dS/f2 where dS is the area of an elemental ring on a detector that senses light scattered over angle q to q+dq, and f is the receiving lens focal length. Substituting,
dP = e-clP0/Ab(q)dAdxdS/f2

But, since any ring detects light scattered at the same scattering angle, dS =2pf f2qdq where f is the fraction of a circle covered by a detector ring. Substituting and integrating, we have the power on ring number i as
Pi=òòòe-clP0/Ab(q)dAdx2p f q dq

Now, if VSF is assumed to be independent of the location of the elementary volume in the water, and if we further assume that VSF is a slowly varying function of angle so that it remains constant over the small angle sub-range, then this is integrated over to:
Pi=e-cll P0pfb(q)[q2i+1–q2i]

Now, the first exponential factor is recognized as optical transmission t. Since there are 32 detectors spanning a radius range 200:1, and detector radii increase logarithmically, if r represents the factor 2001/32 ,
ri = ri-1qmin
so that, we have after substitution and some algebra
bi(q)=[Pi/P0]/[(1-r-2)r2i]/[p l f t q2min]

This is the essential relationship between the power sensed by each silicon ring detector and the VSF averaged over it. All quantities in the above formulation are measured with the LISST-100, though the precise magnitude of the laser power P0 and scattered power Pi requires the responsitivity of the detectors.  Let the photo-detector response be such that the associated post-amplification voltage is:
Vi = Gi Pi, and
V0 = G0 P0,

where G is the gain, (Volts/Watt optical power). Consequently, we have:

bi(q) = G0/Gi Vi/V0/[(1-r-2)r2i]/[p l f t q2min]

The purpose of writing the result in the above form is to show that all quantities are measured, except the responsitivities G. Values of G can be obtained from standards that are traceable to the US National Institute of Standards and Technology, NIST. With a common A/D, the ratio Vi /V0 can be replaced with the ratio of the corresponding digital counts, i.e. Ni/N02.

Thus the task of measuring the VSF reduces to recording the voltages Vi and V0. The LISST-100 instrument records Vi from the 32 ring detectors following an I-V amplifier, using an overall gain of 0.5V/mW-optical3. The corresponding sensitivity for the transmission sensor is ~1mV/mW-optical. As a final form, then, for the assumed value of parameters in this example:
bi(q) = 2.0 10-3Ni/N0/[(1-r-2)r2i]/[p l f  t q2min]